Divided Spheres assumes you have no prior knowledge of spheres except for having played with a beach ball or perhaps noticed the dimple patterns on a golf ball. Every geometric aspect is illustrated, so much so, that you can learn the principles of spherical design simply by following Divided Spheres’ figures. The principles of spherical design and the three main Classes of subdivision plus self-organizing grids based on geometric solids (polyhedra) are explained step-by-step.
New to the Second Edition
- New Foreword by Joseph Clinton, lifelong Buckminster Fuller collaborator
- A new chapter on Self-Organizing Grids by Chris Kitrick on the mathematical techniques for developing optimal single-edge hexagonal tessellations, of varying density, with the smallest edge possible for a particular topology, suggesting ways of comparing their levels of optimization
- An expanded history of the evolution of spherical subdivision
- New applications of spherical design in science, product design, architecture, geographic information systems, virtual reality, the arts and entertainment
- New geodesic algorithms for grid optimization
- New full-color DisplaySphere illustrations to aid readers in visualizing and comparing the various tessellations presented in the book
- Updated Bibliography with references to the most recent advancements in spherical subdivision methods
Here’s what’s inside, Google’s review & some sample pages …
- Chapter 1 – Divided Spheres
- Chapter 2 – Bucky’s Dome
- Chapter 3 – Putting Spheres to Work
- Chapter 4 – Circular Reasoning
- Chapter 5 – Distributing Points
- Chapter 6 – Polyhedral Frameworks
- Chapter 7 – Golf Ball Dimples
- Chapter 8 – Subdivision Schemas
- Chapter 9 – Comparing Results
- Chapter 10 – Self-Organizing Grids
- Appendices
- Stereographic Projection
- Coordinate Rotations
- Geodesic Math
- Bibliography
- Index
- About the Authors
See Google’s review, click here
Sample Pages
 Front Cover |
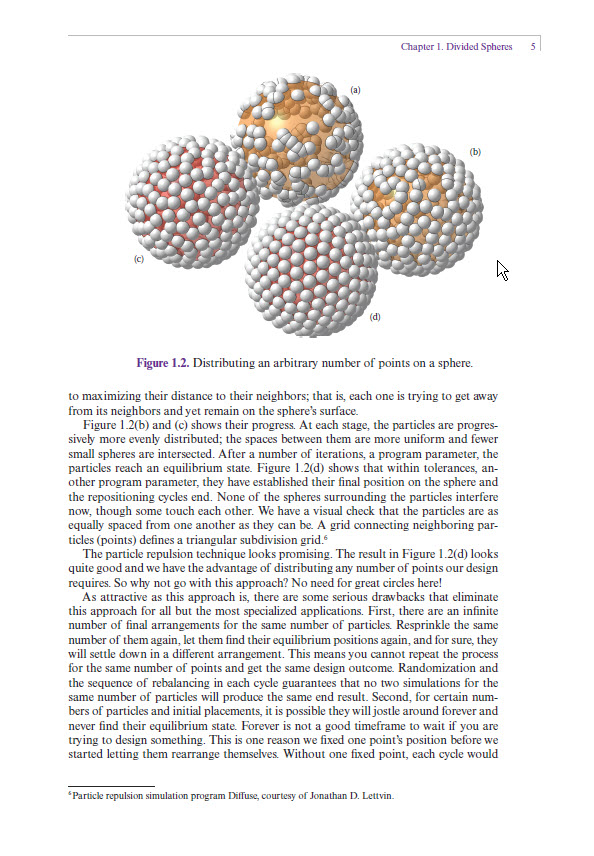 5 5 |
 13 13 |
 25 25 |
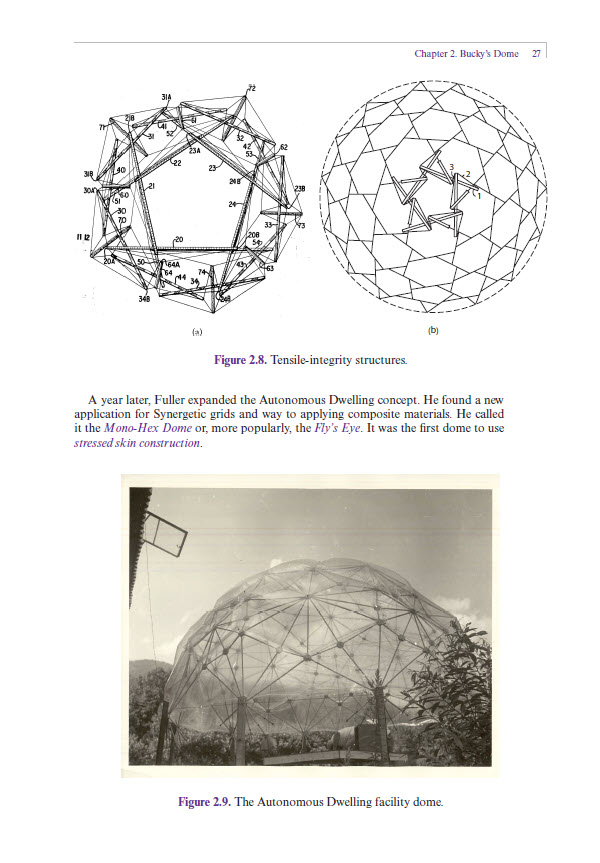 27 27 |
 31 31 |
 82 82 |
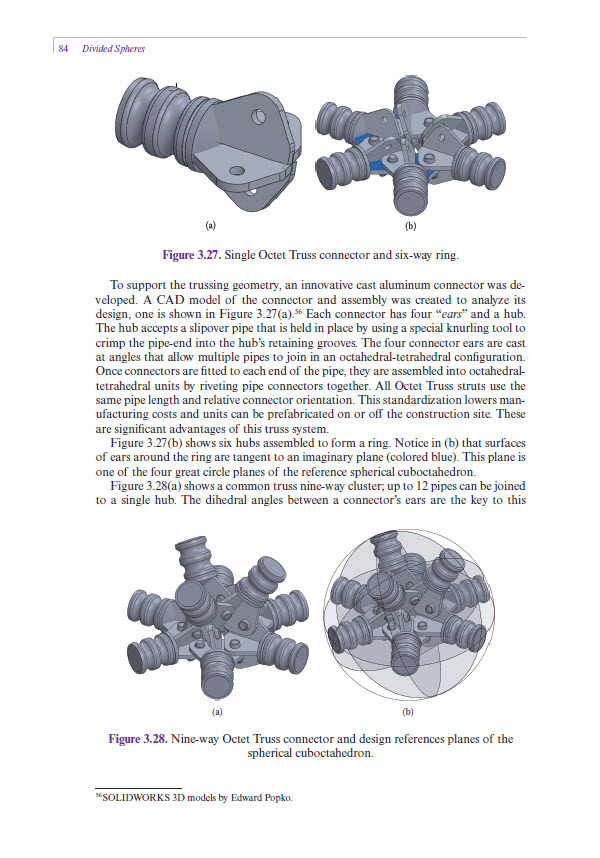 84 84 |
 99 99 |
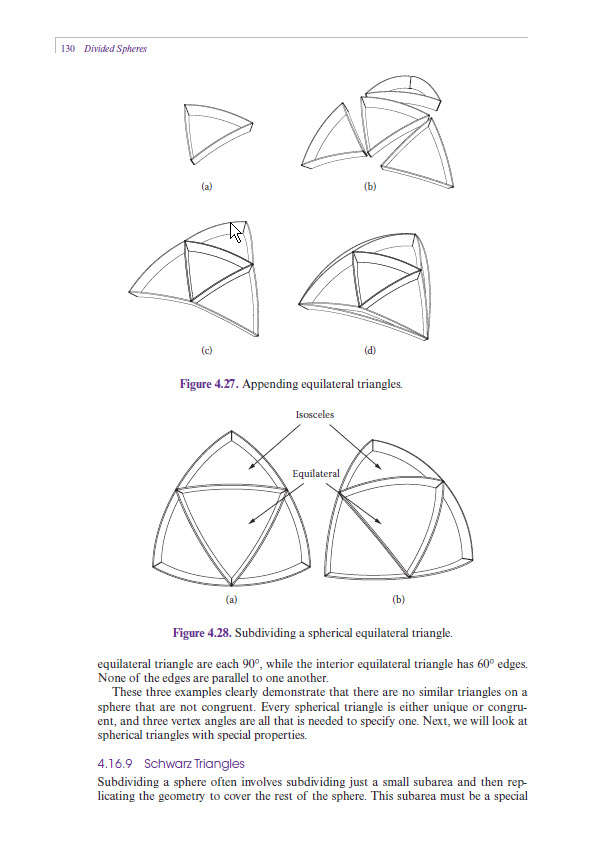 130 130 |
 203 203 |
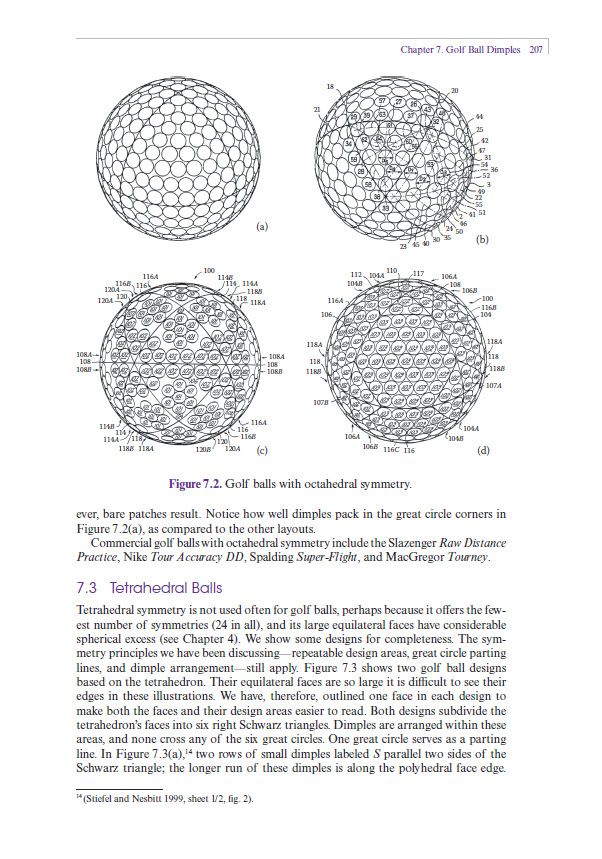 207 207 |
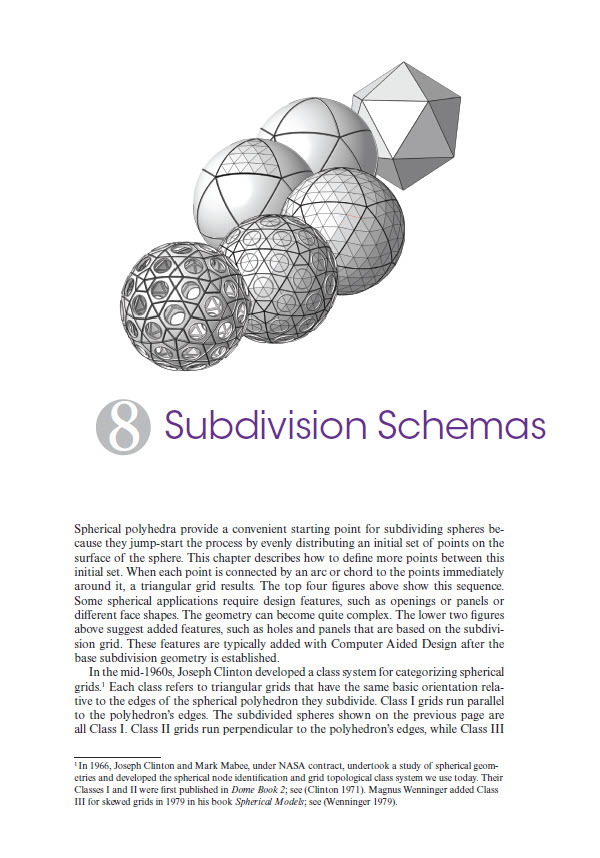 213 213 |
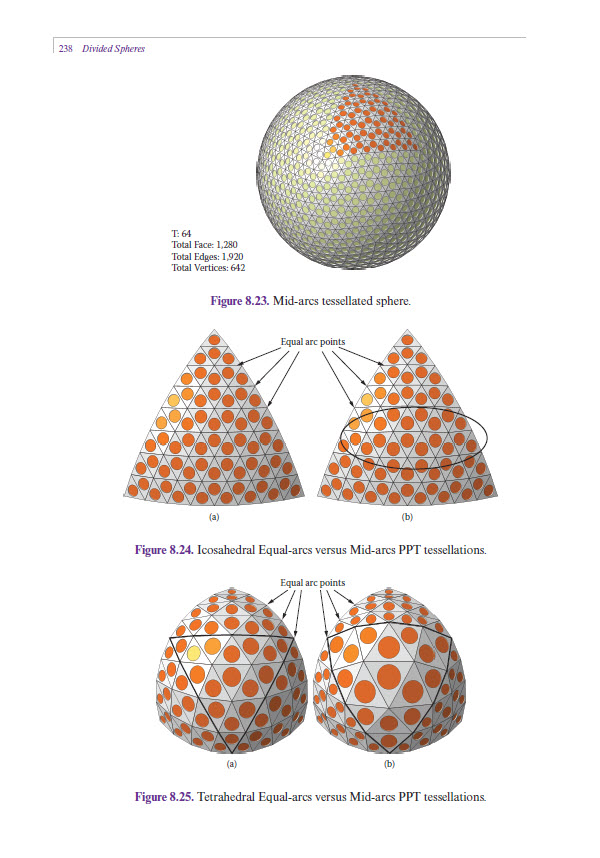 238 238 |
 250 250 |
 265 265 |
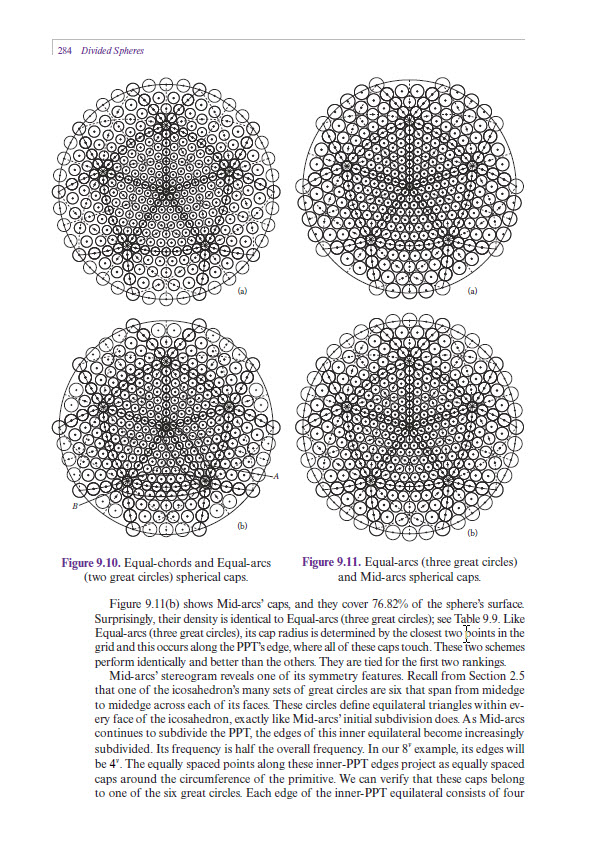 284 284 |
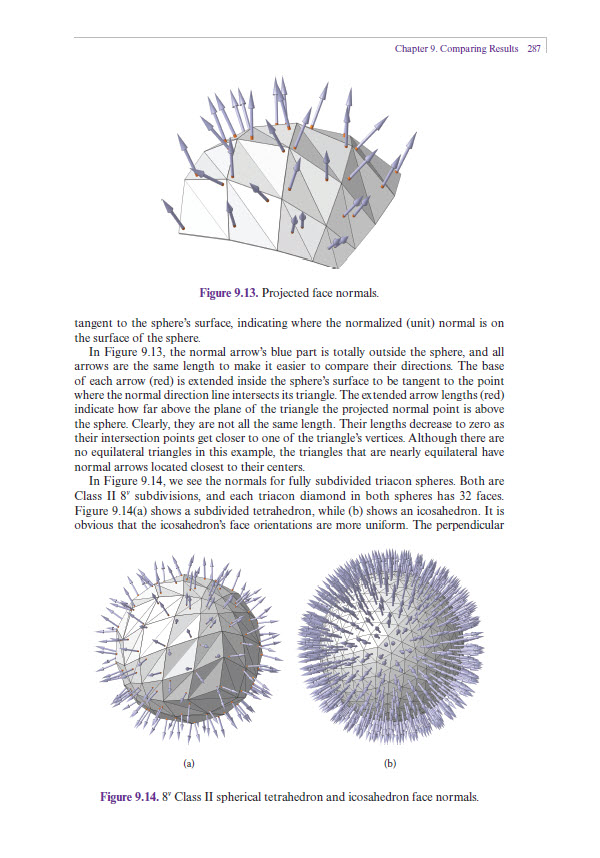 287 287 |
 293 293 |
 326 326 |
 326 326 |
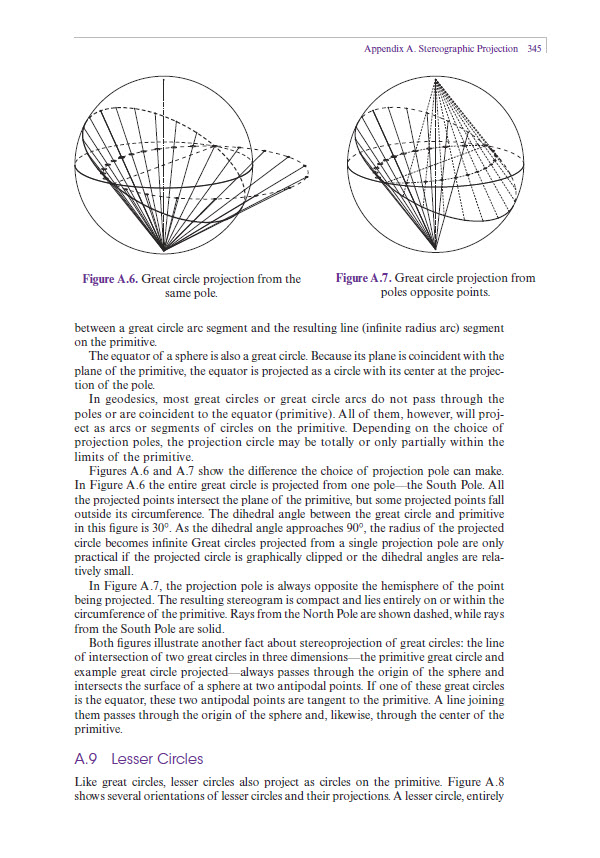 345 345 |
 347 347 |
 Back cover Back cover |
